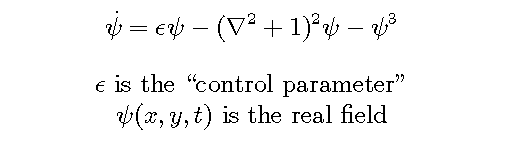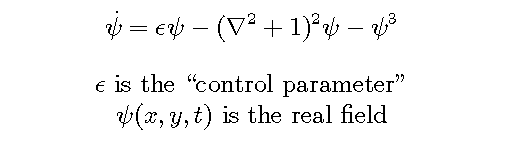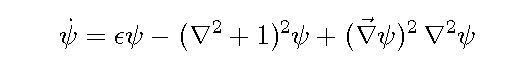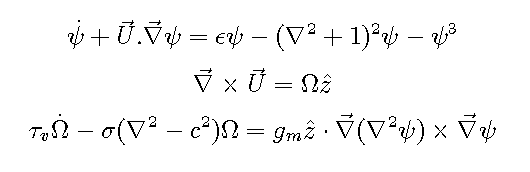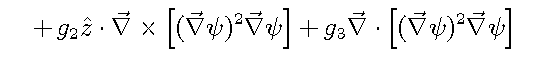Numerical Simulations
We have simulated equations that are simpler than the full fluid and thermal
equations, but that model the phenomenon of an instability to a stripe
state analagous to convection rolls quite well.
The equations we use are based on the Swift-Hohenberg equation
[1]
that was introduced in 1974, and has been much studied since then. It is an
equation for a single real field that is a function of two space variables
(representing the horizontal coordinates in a convection system) and time.
The field can be thought of as the temperature field at the
midplane.
The Swift-Hohenberg eqaution is:
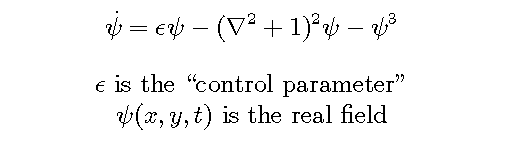
A non-potential model:
For domain coarsening we are particularly interested in the differences between
systems far from equilibrium, and sytems approaching a thermodynamic equilibrium.
The Swift-Hohenberg fails to
model the non-equilibrium nature of the system, because it is "potential". (This means
that the dynamics always decreases a particular functional integral of the field - called
the potential. From
this it can be argued that the dynamics will eventually cease.) A slight modification
yields an equation that is non-potential, so that the dynamics need not be purely
relaxational. The modification changes only the nonlinear piece, to give:
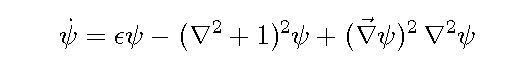
To model the spiral chaos the
Swift-Hohenberg equation is supplemented in the following way:
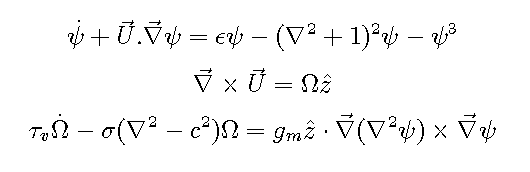
The extra terms include the effect of a slowly varying, horizontal velocity field U that advects the convection rolls
(first equation).
The velocity U is in turn driven by distortions in the rolls, as given through the vertical
vorticity by the second and third equations:
More details can be found in our
paper.
For rotating convection we add the following nonlinear term to the right hand side of the
Swift-Hohenberg equation.
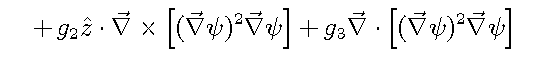
The first term changes sign if the rotation sense is reversed. The second term allows us
to tune the angle at which the patches of stripes grow in.
More details can be found in our
paper [2].
[Return to Home Page]
[Table of Contents]
Last modified Thursday, September 26, 1996
Michael Cross